Baccalauréat S Antilles Guyane 22 juin 2015 - Correction Exercice 1

Page 2 sur 10: Correction Exercice 1

Correction de l'exercice 1 (5 points)

Commun à tous les candidats

Soit $f$ la fonction définie sur l'intervalle $]0~;~+ \infty[$ par $f(x) = \ln x$. Pour tout réel $a$ strictement positif, on définit sur $]0~;~+ \infty[$ la fonction $g_a$ par $g_a(x) = ax^2$. On note $\mathcal{C}$ la courbe représentative de la fonction $f$ et $\Gamma_a$ celle de la fonction $g_a$ dans un repère du plan. Le but de l'exercice est d'étudier l'intersection des courbes $\mathcal{C}$ et $\Gamma_a$ suivant les valeurs du réel strictement positif $a$.

Partie A

On a construit( en annexe 1 à rendre avec la copie ) les courbes $\mathcal{C}$, $\Gamma_{0,05}$, $\Gamma_{0,1}$, $\Gamma_{0,19}$ et $\Gamma_{0,4}$.

1. Nommer les différentes courbes sur le graphique. Aucune justification n'est demandée.

2. Utiliser le graphique pour émettre une conjecture sur le nombre de points d'intersection de$\mathcal{C}$ et $\Gamma_a$ suivant les valeurs (à préciser) du réel $a$.
Il semblerait que :
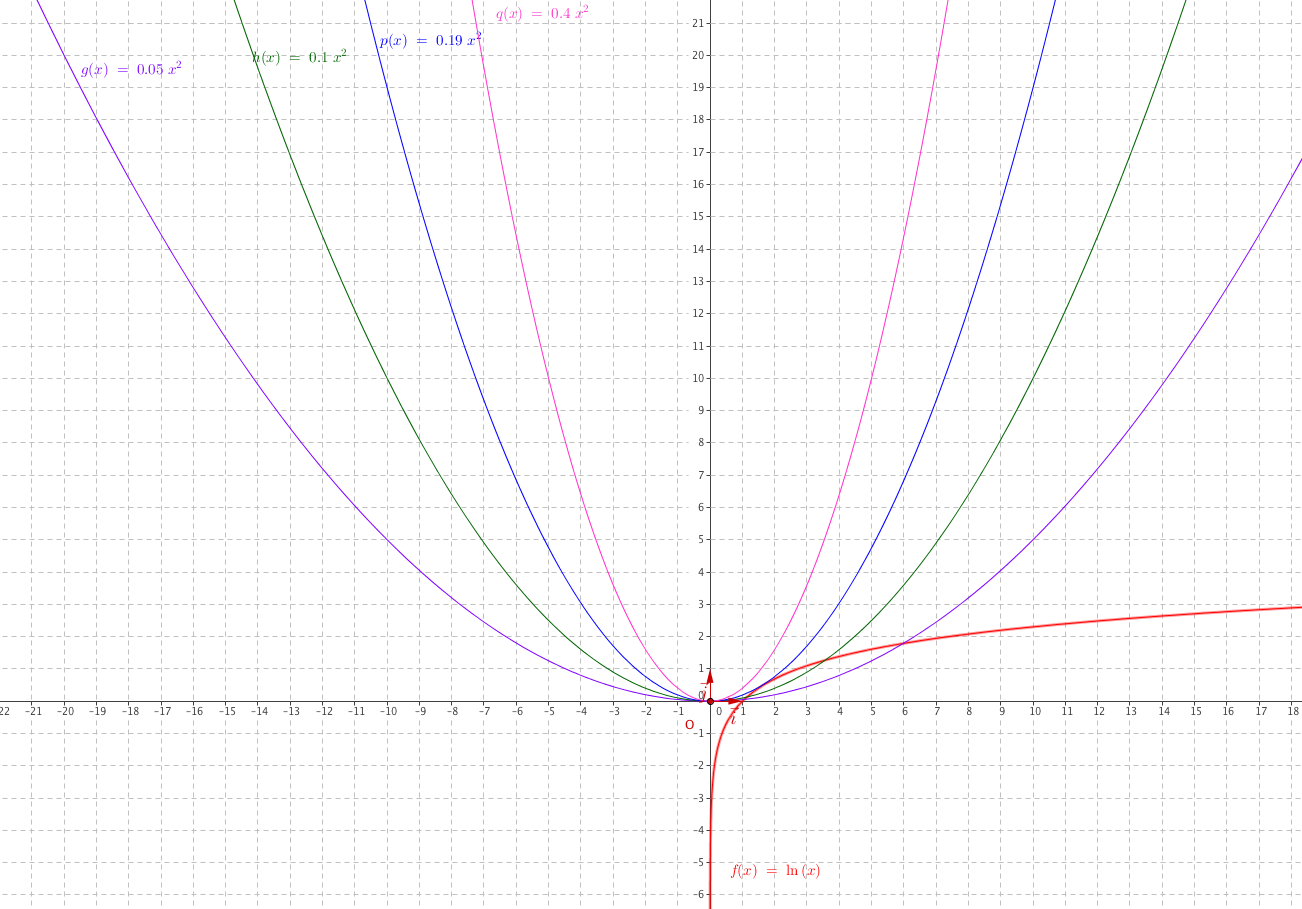
– si $0<a<0,19$, $\mathscr{C}$ et $\Gamma_a$ ont deux points d’intersection
– si $a=0,19$, $\mathscr{C}$ et $\Gamma_a$ ont un point d’intersection
– si $a > 0,19$, $\mathscr{C}$ et $\Gamma_a$ n’ont aucun point d’intersection

Partie B

Pour un réel $a$ strictement positif, on considère la fonction $h_a$ définie sur l'intervalle $]0~;~+ \infty[$ par \[h_a(x) = \ln x - ax^2.\]

1. Justifier que $x$ est l'abscisse d'un point $M$ appartenant à l'intersection de $\mathcal{C}$ et $\Gamma_a$ si et seulement si $h_a (x) = 0.$
Soit $x$ l’abscisse d’un point M appartenant à l’intersection de $\mathscr{C}$ et $\Gamma_a$
On a donc $f(x) = g_a(x) \iff f(x) – g_a(x)=0 \iff \ln x – ax^2 = 0$.

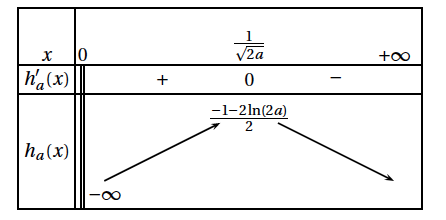
2. 1. On admet que la fonction $h_a$ est dérivable sur $]0~;~+ \infty[$, et on note $h'_a$ la dérivée de la fonction $h_a$ sur cet intervalle. Le tableau de variation de la fonction ha est donné ci-dessous. Justifier, par le calcul, le signe de $h'_a(x)$ pour $x$ appartenant à $]0~;~+ \infty[$.
      
   D’après l »énoncé, $h_a$ est dérivable sur $]0;+\infty[$.
   $h'(x) = \dfrac{1}{x} – 2ax = \dfrac{1 – 2ax^2}{x}$
   $\quad$
   $$\begin{array}{rl} h'(x) \ge 0 &\iff 1 – 2ax^2 \ge 0 \\ & \iff \left(1 – \sqrt{2a}x\right)\left(1 + \sqrt{2a}x\right) \ge 0 \\ & \iff \left(1 – \sqrt{2a}x\right) \ge 0 \quad \text{ car } \left(1 + \sqrt{2a}x\right) > 0 \\\ & \iff 1 \ge \sqrt{2a}x \\ & \iff x \le \dfrac{1}{\sqrt{2a}}\end{array}$$
   Ainsi $h'(a) > 0$ sur $\left]0;\dfrac{1}{\sqrt{2a}} \right[$
   $h'\left(\dfrac{1}{\sqrt{2a}}\right) = 0 $
   $h'(a)<0$ sur $\left]\dfrac{1}{\sqrt{2a}};+\infty\right[$
   $\quad$
   
   2. Rappeler la limite de $\frac{\ln x}{x}$ en $+ \infty$. En déduire la limite de la fonction $h_a$ en $+ \infty$. On ne demande pas de justifier la limite de $h_a$ en $0$.
   $\lim\limits_{x \to +\infty} \dfrac{\ln x}{x} =0$
   $\quad$
   $h_a(x) =x\left(\dfrac{\ln x}{x} – ax\right)$
   $\lim\limits_{x \to +\infty} -ax = -\infty$ car $a> 0$
   Donc $\lim\limits_{x \to +\infty}\left(\dfrac{\ln x}{x} – ax\right) = -\infty$
   Finalement $\left(\dfrac{\ln x}{x} – ax\right) h_a(x) = -\infty$
3. Dans cette question et uniquement dans cette question, on suppose que $a = 0,1$.
   1. Justifier que, dans l'intervalle $\left]0~;~\frac{1}{\sqrt{0,2}}\right]$, l'équation $h_{0,1}(x) = 0$ admet une unique solution. On admet que cette équation a aussi une seule solution dans l'intervalle $]0~;~+ \infty[$.

    

   $$ \newcommand{\mtn}{\mathbb{N}} \newcommand{\mtns}{\mathbb{N}^*} \newcommand{\mtz}{\mathbb{Z}} \newcommand{\mtr}{\mathbb{R}} \newcommand{\mtk}{\mathbb{K}} \newcommand{\mtq}{\mathbb{Q}} \newcommand{\C}{\mathbb{C}} \newcommand{\mch}{\mathcal{H}} \newcommand{\mcp}{\mathcal{P}} \newcommand{\mcb}{\mathcal{B}} \newcommand{\mcl}{\mathcal{L}} \newcommand{\mcm}{\mathcal{M}} \newcommand{\mcc}{\mathcal{C}} \newcommand{\mcmn}{\mathcal{M}} \newcommand{\mcmnr}{\mathcal{M}_n(\mtr)} \newcommand{\mcmnk}{\mathcal{M}_n(\mtk)} \newcommand{\mcsn}{\mathcal{S}_n} \newcommand{\mcs}{\mathcal{S}} \newcommand{\mcd}{\mathcal{D}} \newcommand{\ic}{\text{i}} \newcommand{\mcsns}{\mathcal{S}_n^{++}} \newcommand{\glnk}{GL_n(\mtk)} \newcommand{\mnr}{\mathcal{M}_n(\mtr)} \DeclareMathOperator{\ch}{ch} \DeclareMathOperator{\sh}{sh} \DeclareMathOperator{\th}{th} \DeclareMathOperator{\vect}{vect} \DeclareMathOperator{\card}{card} \DeclareMathOperator{\comat}{comat} \DeclareMathOperator{\imv}{Im} \DeclareMathOperator{\rang}{rg} \DeclareMathOperator{\Fr}{Fr} \DeclareMathOperator{\diam}{diam} \DeclareMathOperator{\supp}{supp} \newcommand{\veps}{\varepsilon} \newcommand{\mcu}{\mathcal{U}} \newcommand{\mcun}{\mcu_n} \newcommand{\dis}{\displaystyle} \newcommand{\croouv}{[\![} \newcommand{\crofer}{]\!]} \newcommand{\rab}{\mathcal{R}(a,b)} \newcommand{\pss}[2]{\langle #1,#2\rangle} \newcommand{\GR}{\mathbb{R}} $$

   D'après le théorème de la bijection :

   • \(\1 \) est une fonction dérivable (donc continue) sur l' intervalle \(I = \left]\2 ; \3\right]\).
   • \(\1\) est strictement croissante sur l' intervalle \(I = \left]\2 ; \3\right]\).
   • \(\lim\limits_{x \to \2}~\1(x)=\4\) et \(\1 \left(\3\right)=\5\)

   \(\1\) réalise donc une bijection de \(\left]\2 ; \3\right]\) sur \(\left]\4;\5\right]\)
   \(\6\in \left]\4;\5\right]\),
   donc l'équation \(\1(x) = \6 \) a une racine unique \(\7\) dans \(\left]\2 ; \3\right]\) .

    

   |-\infty|\frac\{\ln 5 -1\}{2\}|0|\alpha}
   2. Quel est le nombre de points d'intersection de $\mathcal{C}$ et $\Gamma_{0,1}$ ?
L’équation $h_{0,1} = 0$ possède une solution sur chacun des intervalles $\left]0;\dfrac{1}{\sqrt{0,2}}\right]$ et $\left]\dfrac{1}{\sqrt{0,2}};+\infty\right[$.
Ainsi $\mathscr{C}$ et $\Gamma_{0,1}$ ont deux points d’intersection.

4. Dans cette question et uniquement dans cette question, on suppose que $a = \frac{1}{2\text{e}}$.
   1. Déterminer la valeur du maximum de $h_{\frac{1}{2\text{e}}}$.
   $h_{\frac{1}{2\text{e}}}\left(\sqrt{ \text{e}}\right)= \dfrac{-1 – \ln \text{e}^{-1}}{2} = \dfrac{-1 +1}{2} = 0$.
   
   2. En déduire le nombre de points d'intersection des courbes $\mathcal{C}$ et $\Gamma_{\frac{1}{2\text{e}}}$. Justifier.
   La fonction $h_{\frac{1}{2\text{e}}}$ admet donc $0$ comme maximum, atteint seulement en $\dfrac{1}{\sqrt{\dfrac{1}{2\text{e}}}} = \sqrt{\text{e}}$.
   Ainsi $\mathscr{C}$ et $\Gamma_{\frac{1}{2\text{e}}}$ n’ont qu’un seul point d’intersection.
5. Quelles sont les valeurs de $a$ pour lesquelles $\mathcal{C}$ et $\Gamma_{a}$ n'ont aucun point d'intersection ? Justifier.
$\mathscr{C}$ et $\Gamma_a$ n’ont aucun point d’intersection
$$\begin{array}{rl} &\iff \dfrac{-1 – \ln(2a)}{2} <0 \\ &\iff -1 – \ln(2a) < 0 \\ &\iff -\ln(2a) < 1 \\ & \iff \ln(2a) > -1 \\ & \iff 2a > \text{e}^{-1} \\ & \iff a > \dfrac{1}{2\text{e}}\end{array}$$